Practice Exam 1b

[MEEG620]

Problem

Consider in Figure 1 two identical cylinders of radius \(r\) denoted as 1 and 2 in planar motion in a referential 0. Cylinder 1 rolls without sliping along axis \((O, \boldsymbol{\hat{x}}_0)\) of 0. Denote by \(I\) the corresponding contact point. Cylinder 2 rolls and slips along axis \((O, \boldsymbol{\hat{z}}_0)\) of 0. Denote by \(J\) the corresponding contact point. The two cylinders are in contact at point \(K\): assume no-slip at \(K\). Denote by \(\theta\) the (oriented) angle which defines the orientation of the line connecting the centers \(O_1\) and \(O_2\) of the cylinders.

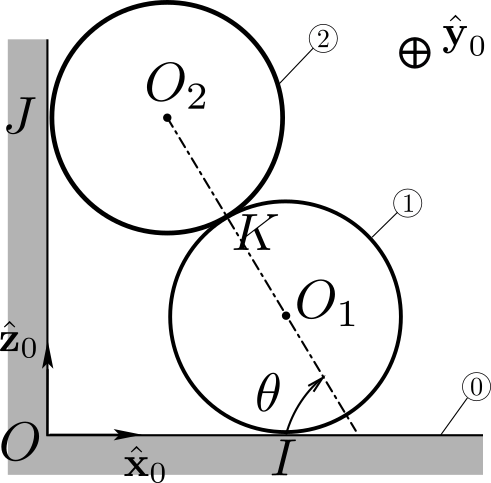
Figure 1
Figure 1

1. Find \(\boldsymbol{r}_{OO_1}\) as a function of \(\theta\). Deduce velocity \(\boldsymbol{v}_{O_1 /0}\) by taking the time-derivative of \(\boldsymbol{r}_{OO_1}\). Then impose no-slip at \(I\) to relate \(\boldsymbol{\omega}_{1/0}\) to \(\dot{\theta}\). Also find \(\boldsymbol{v}_{K\in 1/0}\).

2. Find \(\boldsymbol{r}_{OO_2}\) as a function of \(\theta\). Deduce velocity \(\boldsymbol{v}_{O_2/0}\) by taking the time-derivative of \(\boldsymbol{r}_{OO_2}\). Find \(\boldsymbol{v}_{K\in 2/0}\) in terms of \(\boldsymbol{\omega}_{2/0}\). Now impose no-slip at \(K\) to relate \(\boldsymbol{\omega}_{2/0}\) to \(\dot{\theta}\).

3. Conclude this kinematic study by giving the expressions of the kinematic screws of the two bodies and (relative to ) in terms of \(r\), \(\theta\), and \(\dot{\theta}\).

Note: all derivations can be done on fixed basis \((\boldsymbol{\hat{x}}_0,\boldsymbol{\hat{y}}_0,\boldsymbol{\hat{z}}_0)\).

Formulas:

1. Time-Derivative Formula. Given two rigid bodies (or referentials) \({\cal E}\) and \({\cal F}\), and an arbitrary vector function \(\boldsymbol{U}(t)\), \[ \Big(\frac{d\boldsymbol{U}}{dt} \Big)_{\cal E}=\Big(\frac{d\boldsymbol{U}}{dt} \Big)_{\cal F}+ \boldsymbol{\omega}_{{\cal F}/ {\cal E}}\times \boldsymbol{U} \]

2. Triple product formula: \[ \boldsymbol{A}\times (\boldsymbol{B}\times \boldsymbol{C})= (\boldsymbol{A}\cdot \boldsymbol{C}) \boldsymbol{B}- (\boldsymbol{A}\cdot \boldsymbol{B}) \boldsymbol{C}$ \]

3. Rigid Body Kinematics: Given two rigid bodies (or referentials) \({\cal E}\) and \({\cal F}\), for any two points \(P\) and \(Q\), and at any given time, \[ \boldsymbol{v}_{Q \in {\cal F}/{\cal E}}= \boldsymbol{v}_{P \in {\cal F}/{\cal E}}+ \boldsymbol{\omega}_{{\cal F}/ {\cal E}}\times \boldsymbol{r}_{PQ} \] \[ \boldsymbol{a}_{Q \in {\cal F}/{\cal E}}= \boldsymbol{a}_{P \in {\cal F}/{\cal E}}+ \boldsymbol{\alpha}_{{\cal F}/ {\cal E}}\times \boldsymbol{r}_{PQ} + \boldsymbol{\omega}_{{\cal F}/ {\cal E}}\times (\boldsymbol{\omega}_{{\cal F}/ {\cal E}}\times \boldsymbol{r}_{PQ}) \]

4. Change of Referential Formulas. Given two rigid bodies (or referentials) \({\cal E}\) and \({\cal F}\), for any particle \(P\), and at any given time, \[ \boldsymbol{v}_{P/{\cal E}} = \boldsymbol{v}_{P/{\cal F}} + \boldsymbol{v}_{P\in {\cal F}/ {\cal E}} \] \[ \boldsymbol{a}_{P/{\cal E}} = \boldsymbol{a}_{P/{\cal F}} + \boldsymbol{a}_{P\in {\cal F}/ {\cal E}} + 2 \boldsymbol{\omega}_{{\cal F}/{\cal E}} \times \boldsymbol{v}_{P/{\cal F}} \]

5. Loop formulas: Given 3 rigid bodies \({\cal A}\), \({\cal B}\), \({\cal C}\), and an arbitrary point \(P\), \[ \boldsymbol{\omega}_{{\cal A}/{\cal B}}+ \boldsymbol{\omega}_{{\cal B}/{\cal C}}+ \boldsymbol{\omega}_{{\cal C}/{\cal A}} = \boldsymbol{0} \] \[ \boldsymbol{v}_{P\in {\cal A}/ {\cal B}}+ \boldsymbol{v}_{P\in {\cal B}/ {\cal C}}+ \boldsymbol{v}_{P\in {\cal C}/ {\cal A}}=\boldsymbol{0} \]

Solution

1. We find the velocity of the center \(O_1\) by taking the time-derivative of the position vector \[ \boldsymbol{r}_{OO_1}= (r+2r\cos\theta)\boldsymbol{\hat{x}}_0+ r\boldsymbol{\hat{z}}_0 \] This leads to \[ \boxed{\boldsymbol{v}_{O_1/0} = -2r\dot{\theta}\sin\theta\boldsymbol{\hat{x}}_0} \] We must impose no-slip condition at \(I\): denoting \(\boldsymbol{\omega}_{1/0}= \omega_1 \boldsymbol{\hat{y}}_0\), we find \[ \boldsymbol{v}_{I\in 1/0}= -2r\dot{\theta}\sin\theta\boldsymbol{\hat{x}}_0 +\omega_{1}\boldsymbol{\hat{y}}_0 \times (-r\boldsymbol{\hat{z}}_0) =\boldsymbol{0} \] This gives \[ \boxed{\omega_{1}= -2\dot{\theta}\sin\theta} \] We can now find \(\boldsymbol{v}_{K\in 1/0}\) from \(\boldsymbol{v}_{O_1 /0}\): \[ \boldsymbol{v}_{K\in 1/0} = -2r\dot{\theta}\sin\theta\boldsymbol{\hat{x}}_0 -2\dot{\theta}\sin\theta\boldsymbol{\hat{y}}_0 \times r(-\cos\theta\boldsymbol{\hat{x}}_0+ \sin\theta\boldsymbol{\hat{z}}_0) = - 2r\dot{\theta}\sin\theta( (1+\sin\theta)\boldsymbol{\hat{x}}_0 +\cos\theta\boldsymbol{\hat{z}}_0) \]

Figure 1 1.2. We repeat this work for body 2: from the position vector of \(O_2\) \[ \boldsymbol{r}_{OO_2}=r\boldsymbol{\hat{x}}_0 + (r+2r\sin\theta)\boldsymbol{\hat{z}}_0 \] we find \[ \boxed{\boldsymbol{v}_{O_2 /0}=2r\dot{\theta}\cos\theta\boldsymbol{\hat{z}}_0} \] Note that the directions of the vectors \(\boldsymbol{v}_{O_1/0}\) and \(\boldsymbol{v}_{O_2/0}\) are compatible with the expected motion of the centers. We can find \(\boldsymbol{v}_{K\in 2/0}\) from \(\boldsymbol{v}_{O_2/0}\) and \(\boldsymbol{\omega}_2 =\omega_2 \boldsymbol{\hat{y}}_0\) (\(\omega_2\) is yet to found): \[ \boldsymbol{v}_{K\in 2/0} = 2r\dot{\theta}\cos\theta\boldsymbol{\hat{z}}_0 + \omega_{2}\boldsymbol{\hat{y}}_0 \times r(\cos\theta\boldsymbol{\hat{x}}_0- \sin\theta\boldsymbol{\hat{z}}_0) =-r \omega_{2}\sin\theta\boldsymbol{\hat{x}}_0 + r\cos\theta(2\dot{\theta}- \omega_{2})\boldsymbol{\hat{z}}_0 \] Now we impose the equality \(\boldsymbol{v}_{K\in 2/0} =\boldsymbol{v}_{K\in 1/0}\) to find 2 equations \[ 2\dot{\theta}(1+\sin\theta)= \omega_{2} ,\quad 2\dot{\theta}\sin\theta= (-2\dot{\theta}+ \omega_{2}) \] which give the same solution \[ \boxed{\omega_{2} =2\dot{\theta}(1+\sin\theta)} \]

3. At this point, we have all we need to find the kinematic screws of the 2 bodies \[ \boxed{\{{\cal V}_{1/0}\} = \begin{Bmatrix} \boldsymbol{\omega}_{1/0} \\ \boldsymbol{v}_{I\in 1/0} \end{Bmatrix} =\begin{Bmatrix} -2\dot{\theta}\sin\theta\boldsymbol{\hat{y}}_0 \\ \boldsymbol{0} \end{Bmatrix}_I} \] \[ \boxed{\{{\cal V}_{2/0}\} = \begin{Bmatrix} \boldsymbol{\omega}_{2/0} \\ \boldsymbol{v}_{O_2/0} \end{Bmatrix} = \begin{Bmatrix} 2\dot{\theta}(1+\sin\theta) \boldsymbol{\hat{y}}_0 \\ 2r\dot{\theta}\cos\theta\boldsymbol{\hat{z}}_0 \end{Bmatrix}_{O_2} } \]

Note: Be careful, don’t confuse \(\boldsymbol{v}_{I\in 1/0}\) with \(\boldsymbol{v}_{I/0}\). Similarly, \(\boldsymbol{v}_{K/0}\) is not equal to \(\boldsymbol{v}_{K\in 1/0}=\boldsymbol{v}_{K\in 2/0}\).

© 2024  R. Valéry Roy.